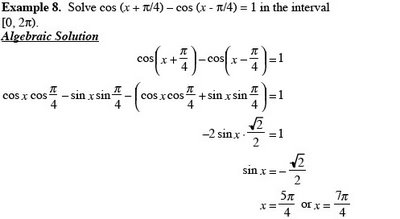No Quickstart, study for test.Chapter 5 TestLessonReview Solving Right Trianglesp.361 #8
To solve a right triangle means to find the measure of each angle and the length of each side.
We do this using the Pythagorean Theorem and right triangle trigonometry.
6.1 Law of SinesI. Introduction To solve an oblique triangle, a triangle with no right angles, we need to be given at least one side and then any other two parts of the triangle.
We can use the Law of Sines or the Law of Cosines.
Today we learn about the Law of Sines.
Law of Sines
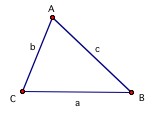
For any triangle ABC with opposite sides a, b, and c
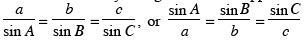
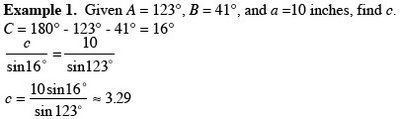
 II. The Ambiguous Case (SSA)
II. The Ambiguous Case (SSA)See p. 430 of the textbook for illustrations
If two sides and one opposite angle are given, three possible situations can occur:
(1) no such triangle exists, (2) one such triangle exists, or (3) two distinct triangles may satisfy the situation.
How do you know what situation you are dealing with?
In the case where the given angle is
acute, the height of the triangle should be calculated.
- If the opposite side is less than the height [a <>
- If the opposite side is equal to the height [a = h] OR the opposite side is greater than the adjacent side [a > b], then only one triangle is possible.
- If the height is less than the opposite side, which is less than the adjacent side [h < a < b], then there are two triangles possible.
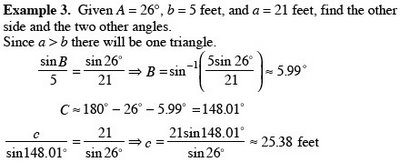











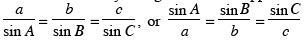

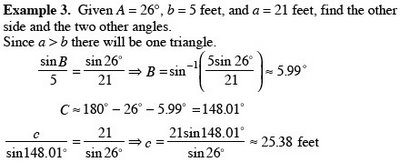


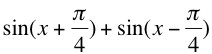 = 1
= 1